Chứng minh:

a) \(\overrightarrow {MN} + \overrightarrow {QE} + \overrightarrow {PQ} + \overrightarrow {NP} = \overrightarrow {ME} \);

b) \(\overrightarrow {AB} + \overrightarrow {MN} + \overrightarrow {BC} + \overrightarrow {CA} + \overrightarrow {PQ} + \overrightarrow {NM} = \overrightarrow {PQ} \);

c) \(\overrightarrow {FK} + \overrightarrow {MQ} - \overrightarrow {FP} - \overrightarrow {MP} + \overrightarrow {QK} = 2\overrightarrow {PK} \);

d) \(\overrightarrow {AD} + \overrightarrow {MP} + \overrightarrow {EK} - \overrightarrow {EP} - \overrightarrow {MD} = \overrightarrow {AK} \).

Lời giải

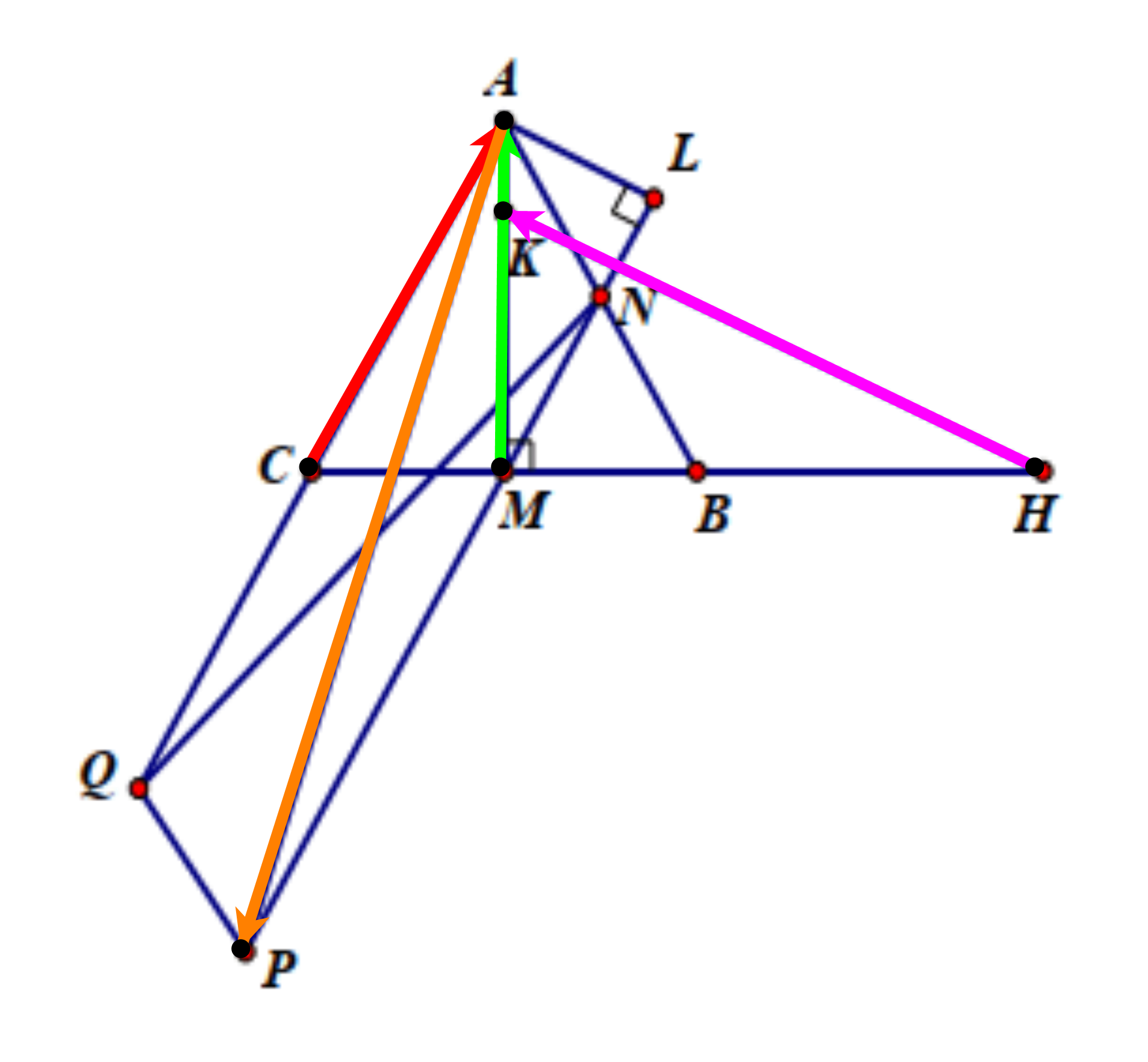
a) Ta có \(\frac{1}{2}\overrightarrow {CB} + \overrightarrow {MA} = \overrightarrow {CM} + \overrightarrow {MA} = \overrightarrow {CA} \) (do M là trung điểm BC).

Vậy \(\left| {\frac{1}{2}\overrightarrow {CB} + \overrightarrow {MA} } \right| = \left| {\overrightarrow {CA} } \right| = CA = a\).

b) Ta có \(\overrightarrow {BA} - \frac{1}{2}\overrightarrow {BC} = \overrightarrow {BA} - \overrightarrow {BM} = \overrightarrow {MA} \) (do M là trung điểm BC).

Tam giác ABC đều cạnh a có M là trung điểm BC.

Suy ra \(CM = BM = \frac{{BC}}{2} = \frac{a}{2}\).

Tam giác ABC đều có AM là đường trung tuyến.

Suy ra AM cũng là đường cao của tam giác ABC.

Tam giác ACM vuông tại M: \(AM = \sqrt {A{C^2} - C{M^2}} = \sqrt {{a^2} - {{\left( {\frac{a}{2}} \right)}^2}} = \frac{{a\sqrt 3 }}{2}\).

Vậy \(\left| {\overrightarrow {BA} - \frac{1}{2}\overrightarrow {BC} } \right| = \left| {\overrightarrow {MA} } \right| = MA = \frac{{a\sqrt 3 }}{2}\).

c) Ta có \(\frac{1}{2}\overrightarrow {AB} + 2\overrightarrow {AC} = \overrightarrow {AN} + \overrightarrow {AQ} \), với N, C là trung điểm AB, AQ.

\( = \overrightarrow {AP} \), với P là đỉnh của hình bình hành AQPN.

Gọi L là hình chiếu của A lên PN.

Ta có MN // AC (MN là đường trung bình của ∆ABC).

Suy ra \(\widehat {ANL} = \widehat {MNB} = \widehat {ACB} = 60^\circ \).

Tam giác ANL vuông tại L:

⦁ \(\sin \widehat {ANL} = \frac{{AL}}{{AN}} \Rightarrow AL = \frac{a}{2}.\sin 60^\circ = \frac{{a\sqrt 3 }}{4}\);

⦁ \(\cos \widehat {ANL} = \frac{{NL}}{{AN}} \Rightarrow NL = \frac{a}{2}.\cos 60^\circ = \frac{a}{4}\).

Ta có PL = PN + NL = AQ + NL = 2AC + NL \( = 2a + \frac{a}{4} = \frac{{9a}}{4}\).

Tam giác ALP vuông tại L: \(AP = \sqrt {A{L^2} + P{L^2}} = \sqrt {{{\left( {\frac{{a\sqrt 3 }}{4}} \right)}^2} + {{\left( {\frac{{9a}}{4}} \right)}^2}} = \frac{{a\sqrt {21} }}{2}\).

Vậy \(\left| {\frac{1}{2}\overrightarrow {AB} + 2\overrightarrow {AC} } \right| = \left| {\overrightarrow {AP} } \right| = AP = \frac{{a\sqrt {21} }}{2}\).

d) Gọi K là điểm nằm trên đoạn AM thỏa mãn \(MK = \frac{3}{4}MA\)và H là điểm thuộc tia MB sao cho MH = 2,5MB.

Khi đó \(\overrightarrow {MK} = \frac{3}{4}\overrightarrow {MA} ,\,\,\overrightarrow {MH} = 2,5\overrightarrow {MB} \).

Ta có \(\frac{3}{4}\overrightarrow {MA} - 2,5\overrightarrow {MB} = \overrightarrow {MK} - \overrightarrow {MH} = \overrightarrow {HK} \).

Ta có \(MK = \frac{3}{4}MA = \frac{3}{4}.\frac{{a\sqrt 3 }}{2} = \frac{{3a\sqrt 3 }}{8}\) và \(MH = 2,5MB = 2,5.\frac{a}{2} = \frac{{5a}}{4}\).

Tam giác KMH vuông tại M: \(HK = \sqrt {M{K^2} + M{H^2}} = \sqrt {{{\left( {\frac{{3a\sqrt 3 }}{8}} \right)}^2} + {{\left( {\frac{{5a}}{4}} \right)}^2}} = \frac{{a\sqrt {127} }}{8}\).

Lời giải

a) \(P = \frac{{{x^2} + x}}{{{x^2} - 2x + 1}}:\left( {\frac{{x + 1}}{x} - \frac{1}{{1 - x}} + \frac{{2 - {x^2}}}{{{x^2} - x}}} \right)\)

\( = \frac{{x\left( {x + 1} \right)}}{{{{\left( {x - 1} \right)}^2}}}:\left[ {\frac{{x + 1}}{x} + \frac{1}{{x - 1}} + \frac{{2 - {x^2}}}{{x\left( {x - 1} \right)}}} \right]\)

\( = \frac{{x\left( {x + 1} \right)}}{{{{\left( {x - 1} \right)}^2}}}:\left[ {\frac{{\left( {x + 1} \right)\left( {x - 1} \right) + x + 2 - {x^2}}}{{x\left( {x - 1} \right)}}} \right]\)

\( = \frac{{x\left( {x + 1} \right)}}{{{{\left( {x - 1} \right)}^2}}}:\frac{{x + 1}}{{x\left( {x - 1} \right)}} = \frac{{x\left( {x + 1} \right)}}{{{{\left( {x - 1} \right)}^2}}}.\frac{{x\left( {x - 1} \right)}}{{x + 1}} = \frac{{{x^2}}}{{x - 1}}\).

b) Ta có \(P < 1 \Leftrightarrow \frac{{{x^2}}}{{x - 1}} < 1\)

\[ \Leftrightarrow \frac{{{x^2}}}{{x - 1}} - 1 < 0 \Leftrightarrow \frac{{{x^2} - x + 1}}{{x - 1}} < 0\]

\[ \Leftrightarrow \frac{{{{\left( {x - \frac{1}{2}} \right)}^2} + \frac{3}{4}}}{{x - 1}} < 0 \Leftrightarrow x - 1 < 0\] (vì \[{\left( {x - \frac{1}{2}} \right)^2} + \frac{3}{4} \ge \frac{3}{4} > 0,\,\,\forall x \in \mathbb{R}\]).

⇔ x < 1.

Vậy x < 1 thì P < 1.

c) Vì x > 2 nên x – 2 > 0.

Do đó x – 1 > x – 2 > 0.

Ta có \(P = \frac{{{x^2}}}{{x - 1}} = \frac{{{x^2} - 1 + 1}}{{x - 1}} = x + 1 + \frac{1}{{x - 1}} = x - 1 + \frac{1}{{x - 1}} + 2\).

Áp dụng bất đẳng thức Cauchy, ta có: \(x - 1 + \frac{1}{{x - 1}} \ge 2\sqrt {\frac{{x - 1}}{{x - 1}}} = 2\sqrt 1 = 2,\,\forall x > 2\).

\( \Leftrightarrow x - 1 + \frac{1}{{x - 1}} + 2 \ge 2 + 2 = 4\).

⇔ P ≥ 4.

Dấu “=” xảy ra ⇔ (x – 1)² = 1 ⇔ x – 1 = 1 hoặc x – 1 = –1.

⇔ x = 2 (loại vì x > 2) hoặc x = 0 (loại vì x > 2).

Vậy P không có giá trị nhỏ nhất khi x > 2.